Secondary Quantum Number
Magnetic Quantum Number
(n)
Shell
(l)
SubShell
(ml)
Orbital









Quantum Numbers
The Principal Quantum Number, n
The first quantum number, given the symbol n, is of primary importance in determining the energy of an electron. The energy of each electron depends mainly, but not completely, upon the value of n. As n increase, the energy of the electron increases and, on the average, it is found further out from the nucleus. The quantum number n can take on only intergral values, starting with 1:
n = 1, 2, 3, 4,…
An electron for which n = 1 is said to be in the first principle level. The value principle quantum number can also be referred to as the shell.
The Secondary Quantum Number, l
Each principle energy level includes one or more sublevels. The sublevels are denoted by the second quantum number, l. As we will see later, the general shape of the electron cloud associated with an electron is determine by l. Larger values of l produce more complex shapes. The quantum numbers n and l are related: l can take on any intergral value starting with 0 and going up to a maximum of (n – 1). That is,
l = 0, 1, 2,…(n-1)
Another method is commonly used to designate sublevels. Instead of giving the quantum number l, the letters s, p, d, or f indicate the sublevels l = 0, 1, 2, and 3 respectively.
The secondary quantum number, l, eventually relates energy levels to the shape of the electron orbitals. It will also help to explain the regions of the periodic table
| Sublevels for the First Four Principle Quantum Levels | ||||
| n | 1 | 2 | 3 | 4 |
| l | 0 | 0 1 | 0 1 2 | 0 1 2 3 |
| s | s p | s p d | s p d f | |
These letters come from the adjectives used by spectroscopists to describe spectral lines: sharp, principal, diffuse, and fundamental.
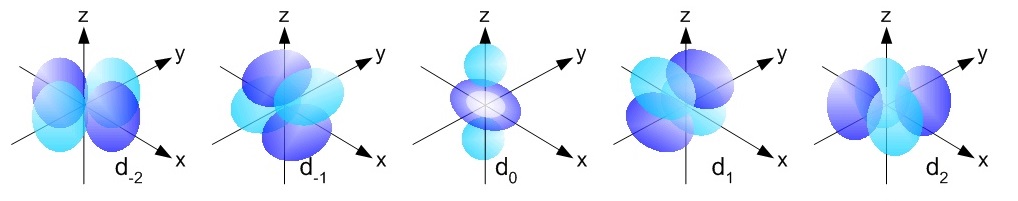
The Magnetic Quantum Number, ml
Each sublevel contains one or more orbitals, which differ from one another in the value assigned to the third quantum number, ml. This quantum number determines the direction in space of the electron cloud surrounding the nucleus. The value of ml can have an integral value, including 0 between - l and + l, that is
ml = – l,…,-1, 0, +1,…,+ l
The maximum value of ml depends on the value of l. For example, when l = 1, which designates the p subshell, there are three permissible values of ml: = –1, 0, and +1. Thus, three distinct regions of space, called atomic orbitals, are associated with a p subshell. We refer to these orbitals as the px, py, and pz orbitals.
The Spin Quantum Number, ms
The final (fourth) quantum number was added to account for two kinds of evidence – some additional spectral line-splitting in a magnetic field and different kinds of magnetism.
In 1925, Pauli, suggested that each electron spins on its axis. He suggested not only that a spinning electron is like a tiny magnet, but that it could have only two spins. Using an analogy, we can say an electron is like a spinning top, which can spin either clockwise or counterclockwise. For an electron, the two spins are equal in magnitude but opposite in direction, and these are the only choices; i.e., the spin is quantized to two and only two values. This fourth quantum number is called the spin quantum number, ms, and is given values of either – ½ or + ½. Qualitatively, we refer to the spin as either clockwise or counterclockwise or as up or down.
This lead to the Pauli Exclusion Principle, which states that no two electrons can have the same set of quantum numbers, and therefore the spin quantum number is added.
Creating the Four Quantum Numbers
Key Experimental Work |
Theoretical Explanation |
Quantum Theory |
low-resolution line spectra |
principal quantum number, n |
All electrons in all atoms can be described by four quantum numbers |
high-resolution line spectra |
secondary quantum number, l |
|
spectra in magnetic field |
magnetic quantum number, ml |
|
ferro- and parramagnetism |
spin quantum number, ms |
Permissible Values of the Quantum Numbers
Secondary Quantum Number |
Magnetic Quantum Number |
Spin Quantum Number | Number of Electrons in Subshel | Number of Electrons in Energy Level | |||
(n) |
(l) |
Type | Kind | (ml) |
(ms) | ||
| 1 | 0 | s | 1s |  |
- ½, +½ | 2 | 2 |
| 2 | 1 | s | 2s |  |
- ½, +½ | 2 | 8 |
| 0 | p | 2p |  |
- ½, +½ for all values of ml | 6 | ||
| 3 | 0 | s | 3s |  |
- ½, +½ | 2 | 18 |
| 1 | p | 3p |  |
- ½, +½ for all values of ml | 6 | ||
| 2 | d | 3d |  |
- ½, +½ for all values of ml | 10 | ||
| 4 | 0 | s | 4s |  |
- ½, +½ | 2 | 32 |
| 1 | p | 4p |  |
- ½, +½ for all values of ml | 6 | ||
| 2 | d | 4d |  |
- ½, +½ for all values of ml | 10 | ||
| 3 | f | 4f |  |
- ½, +½ for all values of ml | 14 | ||
| 5 | 0 | s | 5s | - ½, +½ | 2 | 50 | |
| 1 | p | 5p | - ½, +½ for all values of ml | 6 | |||
| 2 | d | 5d | - ½, +½ for all values of ml | 10 | |||
| 3 | f | 5f | - ½, +½ for all values of ml | 14 | |||
| 4 | g | 5g | - ½, +½ for all values of ml | 18 | |||